By Katie Mooney
Considering the complexity of our architectural world, sound construction seems nearly impossible without the mathematical tools that allow for exact measurement and analysis before the first bricks are laid. Yet throughout history, different civilizations have built monuments, temples, and other grand public spaces with little to no mathematical and architectural instruments or written guidance. Full understanding and articulation of these principles almost always came years, indeed centuries, after the construction of ancient edifices. The structural feats of early builders is one of the central themes explored in the recently published book Mathematical Excursions to the World’s Great Buildings, winner of the 2012 PROSE Award in the Architecture and Urban Planning category. The author is Alexander Hahn, professor of mathematics at the University of Notre Dame and Phi Beta Kappa member. Each chapter takes readers on a historical tour of structures from different eras, highlighting the progressive use of elementary mathematics. The adventure begins with a glimpse into the prehistoric understanding and application of fundamental shapes and organization.
The earliest humans were aware of spatial relationships and used geometry to lay out their shelters. They observed the forms and structures of nature, gained a sense of basic shapes and numbers, and then (subconsciously or not) used what they observed to organize their existence, for instance, in the circular or rectangular huts that they constructed. But not all early structures were erected for practical purposes. Some of the most complex projects of ancient civilizations were motivated by the need to create visual expression of the power of rulers and gods. Perhaps the best examples are the great pyramids of Giza, built as burial tombs during the fourth dynasty (c. 2575–c. 2465 B.C.) for pharaohs Khufu, Khafre, and Menkaure. Using ramps, levers, sturdy rope, and no metal harder than copper, Egyptian laborers precisely cut large blocks of stone and stacked them in on top of one another, forming spectacular monuments that displayed the grandeur of the powerful.
Thousands of year later, between 600 B.C. and A.D. 200, Greece and its colonies along the Mediterranean made major advances in mathematics and science. A new rational approach was applied to building and architecture as reason (logos) began to replace the notion that gods and demi-gods controlled nature (mythos). This sense of logic and discipline was exhibited in the geometric forms of Greek architecture. The Greeks constructed buildings by tying ropes to pegs stuck in the ground and stretching these ropes to create straight lines and circular arcs. They later abstracted this practice in their development of geometry. However, scholars still debate whether Greek architects adhered to strict mathematical and geometrical relationships in their designs.
For example, consider the Parthenon, the famous Greek temple completed in 438 B.C. on the Athenian acropolis in honor of the city’s patron goddess, Athena. Today mathematicians can easily draw a connection between the façade of the Parthenon structure and the notion of the golden rectangle. A golden rectangle is any rectangle whose side lengths are in the “golden ratio” to each other (approximately 1 to 1.618). The distinctive feature of a golden rectangle is that if the largest square that it contains is removed, the remaining area is another golden rectangle. Using a sequence of this process it is possible to place a series of golden rectangles of diminishing sizes on the façade of the Parthenon that fit its basic features reasonably well (see figure below). But did the Greeks use golden rectangles as a template, or did later scholars simply superimpose them on the façade? Despite the approximate “fit” of the golden rectangles onto the façade of the Parthenon, there is no conclusive evidence that precise geometric ratios were intentionally employed in Greek designs.
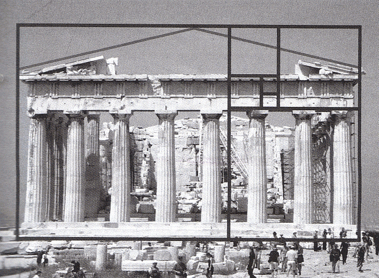
Parthenon showing the golden rectangle. Courtesy Princeton University Press.
Roman civilization flourished alongside the Greek civilization from 600 B.C. to A.D. 400. Not surprisingly, Greek architecture also influenced Roman builders who were busy designing harbors, docks, amphitheaters, aqueducts, and stadiums. Shared features in Greek and Roman construction included vertical columns, horizontal elements, and triangular components, but Roman structures also made extensive use of arches, vaults, and domes. For instance, the Colosseum is a composition of rows of arches and configurations of barrel vaults that provide entry and support the seating sections. While Roman builders knew that their edifices would have to withstand the thrusts and stresses of aches and domes, they did not know how to deal with forces mathematically. They did not know how to regard forces as vectors that can be quantified and split into components. Instead, they built primarily by trial and error, copying designs that had been successful in the past. Sometimes the properties of building materials were brilliantly manipulated in order to make structures more stable. The Pantheon is a case in point. Completed in A.D. 128, its builders used concrete made with light volcanic pumice to make the upper sections of the dome less heavy, thereby reducing the thrusts placed on the lower portions of the structure.
From Egypt to Greece to Rome, breathtaking edifices were created with limited knowledge of the underlying mathematics. As modern scholars continue explore historical architecture using refined mathematical principles, they uncover striking examples of the intuition and accuracy of early architects and builders. In our age of digital building plans and computerized construction, it is often forgotten that the most prized ancient spaces are upheld, quite literally, by simple applications of algebra, geometry, trigonometry, and calculus.
Katie Mooney is a 2012 Phi Beta Kappa graduate of the University of Notre Dame where she majored in psychology and pre-medical studies. Notre Dame is home to the Epsilon of Indiana chapter of Phi Beta Kappa.




